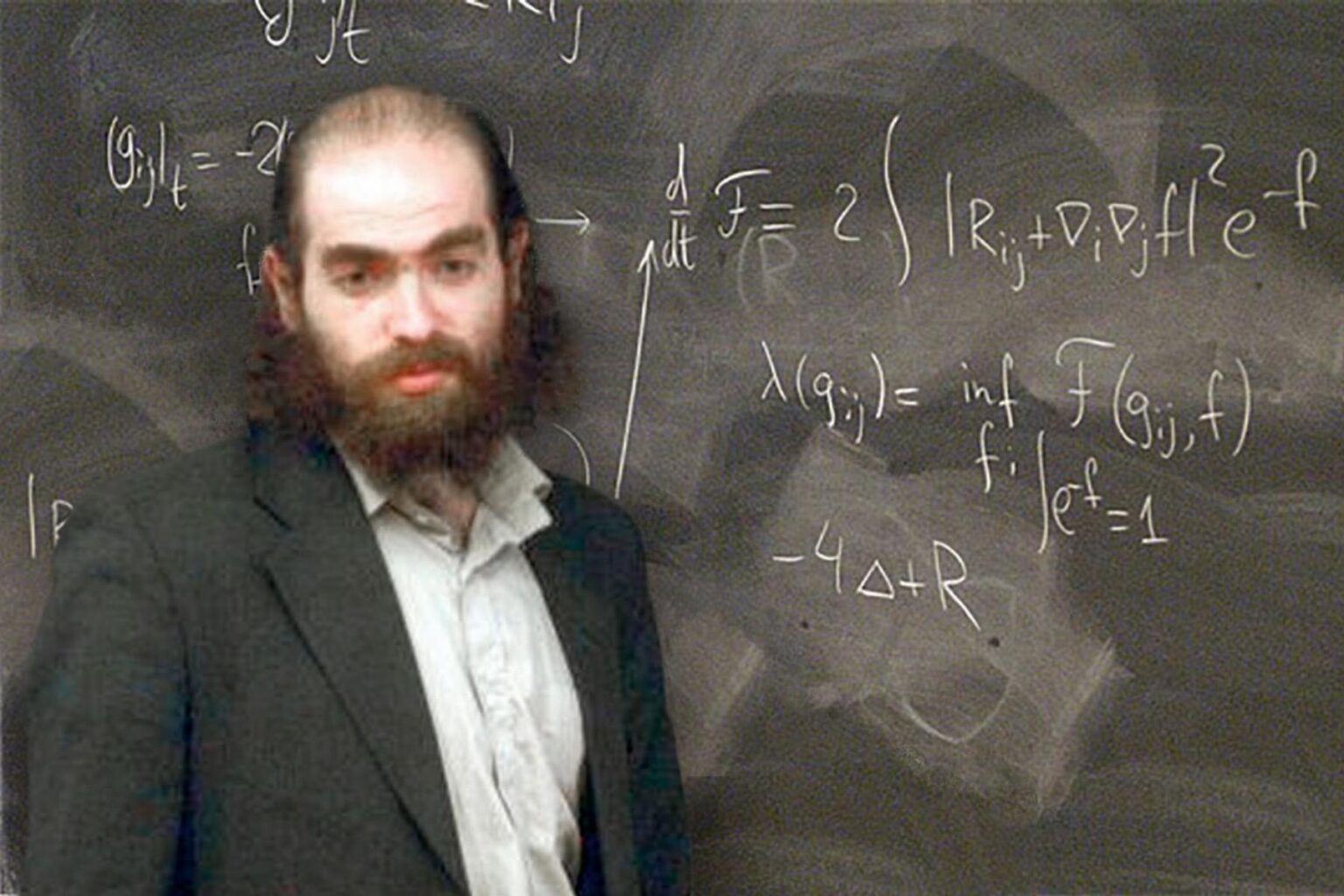
In the annals of mathematical history, few stories are as compelling as that of Grigori Perelman, a Russian mathematician whose intellectual prowess and unwavering principles challenged conventional notions of success.
His groundbreaking solution to the Poincaré Conjecture not only resolved a century-old mathematical mystery but also exposed a profound narrative of genius that transcends monetary recognition. Perelman’s remarkable journey from a reclusive scholar in St. Petersburg to a globally acknowledged mathematician raises provocative questions about the true value of intellectual achievement and the complex relationship between brilliance, fame, and personal integrity.
Briefly keys
- Grigori Perelman solved the century-old Poincaré Conjecture, a complex mathematical problem that eluded mathematicians for decades.
- He rejected the prestigious Millennium Prize of $1 million, demonstrating extraordinary intellectual integrity and indifference to financial rewards.
- Perelman developed groundbreaking mathematical techniques using the Ricci flow method to transform understanding of three-dimensional topological spaces.
- A mathematical prodigy from Leningrad, he achieved a perfect score at the International Mathematical Olympiad at age 16.
- Despite his revolutionary contributions to geometric topology, Perelman chose a reclusive lifestyle, prioritizing personal values over public recognition.
A Mathematical Genius From Leningrad
A prodigy’s journey begins in the historic city of Leningrad, where Grigori Perelman first revealed his extraordinary mathematical potential. Engaged in the rich intellectual culture of Soviet Leningrad, young Perelman was nurtured by parents who understood the transformative power of education. His mother, a mathematics teacher, and his father, an electrical engineer, cultivated an environment that celebrated intellectual curiosity and problem-solving.
At just ten years old, Perelman’s mathematical upbringing took a decisive turn when he joined Sergei Rukshin’s mathematics club, marking the early stages of his remarkable trajectory. The Leningrad Special Mathematics and Physics School became his intellectual crucible, where his innate brilliance was honed and refined.
By age 16, Perelman’s mathematical prowess was undeniable, culminating in a perfect score at the International Mathematical Olympiad, signaling the emergence of a true mathematical genius. His early academic success at Leningrad State University under influential mathematicians like Viktor Zalgaller further solidified his path towards becoming a world-renowned mathematical prodigy.
Cracking the Poincaré Conjecture

Unraveling the mathematical mystery that had puzzled mathematicians for nearly a century, Grigori Perelman’s groundbreaking solution to the Poincaré Conjecture stands as a proof of human intellectual perseverance.
His revolutionary approach centered on the Ricci flow method, transforming geometric understanding by elegantly proving the Poincaré significance of three-dimensional topological spaces. By systematically addressing complex mathematical challenges, Perelman demonstrated how continuous deformations could disclose fundamental truths about manifold structures.
International mathematical community widely acknowledged the profound significance of solving a century-old geometric problem that had eluded mathematicians for decades.
Working meticulously, he published three seminal preprints that would fundamentally reshape geometric topology. His proof not only solved a century-old problem but also disclosed profound insights into the intricate world of mathematical spaces.
Mathematicians worldwide celebrated his achievement, recognizing how Perelman’s innovative techniques transcended traditional mathematical boundaries and opened new pathways of geometric exploration.
Awards and Accolades Rejected

Following his groundbreaking solution to the Poincaré Conjecture, Grigori Perelman shocked the mathematical world by systematically rejecting prestigious awards that typically represent the highest honor in academic mathematics.
When prestigious awards came calling, Perelman stunned the academic world by systematically declining them. The Fields Medal – mathematics’ highest honor – and the $1 million Millennium Prize were both rejected. His rationale transcended monetary value, reflecting a profound commitment to intellectual integrity that challenged conventional notions of academic recognition.
His actions sparked widespread debate, with some viewing his choices as principled eccentricity and others seeing them as a provocative critique of academic recognition systems. Living with his mother in a modest St. Petersburg apartment, Perelman maintained his radical stance of complete detachment from academic and social recognition.
The Reclusive Mathematician’s Path
Although mathematical prodigies often emerge from supportive academic environments, Grigori Perelman’s trajectory defied conventional expectations. Raised by a mathematics teacher mother who sacrificed her own academic career, Perelman’s early academic influences were deeply rooted in intellectual rigor.
His mother’s dedication and the specialized education at Leningrad Secondary School #239 cultivated his extraordinary mathematical talents from a young age. Sergei Rukshin’s mathematics training program further nurtured Perelman’s remarkable abilities, enabling him to achieve a perfect score at the International Mathematical Olympiad.
His unconventional path continued through groundbreaking research in Riemannian geometry, where he solved complex mathematical problems that had challenged experts for decades. Despite his incredible achievements, Perelman chose a path of intellectual isolation, retreating from academic recognition and living a secluded life in Saint Petersburg, prioritizing personal integrity over professional acclaim.
Proving the Poincaré conjecture not only solidified his mathematical genius but also demonstrated his unparalleled ability to solve century-old mathematical problems that had stumped generations of mathematicians.
Legacy Beyond Monetary Rewards
The essence of mathematical brilliance often transcends financial compensation, a truth epitomized by Grigori Perelman’s remarkable journey. His intellectual humility shines through his deliberate rejection of prestigious awards and monetary prizes, demonstrating that true scientific achievement is rooted in pure intellectual pursuit rather than external validation.
Perelman’s groundbreaking work on the Poincaré conjecture and Thurston’s geometrization conjecture represents an enduring influence that extends far beyond monetary rewards, reshaping our understanding of geometric topology and Riemannian geometry. His meticulous proofs and innovative techniques continue to inspire mathematicians worldwide, setting unprecedented standards of mathematical rigor.
By prioritizing mathematical truth over personal acclaim, Perelman transformed complex mathematical challenges into watershed moments of scientific discovery, leaving an indelible mark on the mathematical landscape that resonates through generations of researchers. His profound contributions were particularly significant during his research at Steklov Institute, where he advanced critical mathematical understanding before his academic withdrawal in 2003.
Wrapping up
Perelman’s extraordinary journey transcends mathematical achievement. By rejecting prestigious awards and prioritizing intellectual integrity, he redefined success beyond monetary metrics. His groundbreaking solution to the Poincaré Conjecture represents more than mathematical brilliance—it symbolizes unwavering commitment to pure knowledge. The legacy of this remarkable mathematician serves as an enduring testament to the power of intellectual curiosity and principled scholarship.